binary-tree-maximum-path-sum

< Previous 　　　　　　　　　　　　　　　　 Next >

124. Binary Tree Maximum Path Sum (Hard)

A path in a binary tree is a sequence of nodes where each pair of adjacent nodes in the sequence has an edge connecting them. A node can only appear in the sequence at most once. Note that the path does not need to pass through the root.

The path sum of a path is the sum of the node's values in the path.

Given the root of a binary tree, return the maximum path sum of any non-empty path.

 

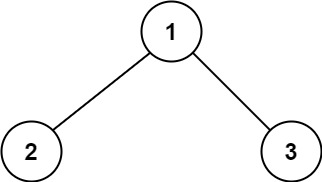
Example 1:

Input: root = [1,2,3]
Output: 6
Explanation: The optimal path is 2 -> 1 -> 3 with a path sum of 2 + 1 + 3 = 6.

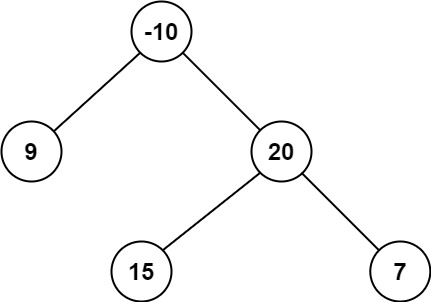
Example 2:

Input: root = [-10,9,20,null,null,15,7]
Output: 42
Explanation: The optimal path is 15 -> 20 -> 7 with a path sum of 15 + 20 + 7 = 42.

 

Constraints:

• The number of nodes in the tree is in the range [1, 3 * 104].
• -1000 <= Node.val <= 1000

Related Topics

[Dynamic Programming] [Tree] [Depth-First Search] [Binary Tree]

Similar Questions

1. Path Sum (Easy)
2. Sum Root to Leaf Numbers (Medium)
3. Path Sum IV (Medium)
4. Longest Univalue Path (Medium)
5. Time Needed to Inform All Employees (Medium)