number-of-ways-to-paint-n-3-grid

< Previous 　　　　　　　　　　　　　　　　 Next >

1411. Number of Ways to Paint N × 3 Grid (Hard)

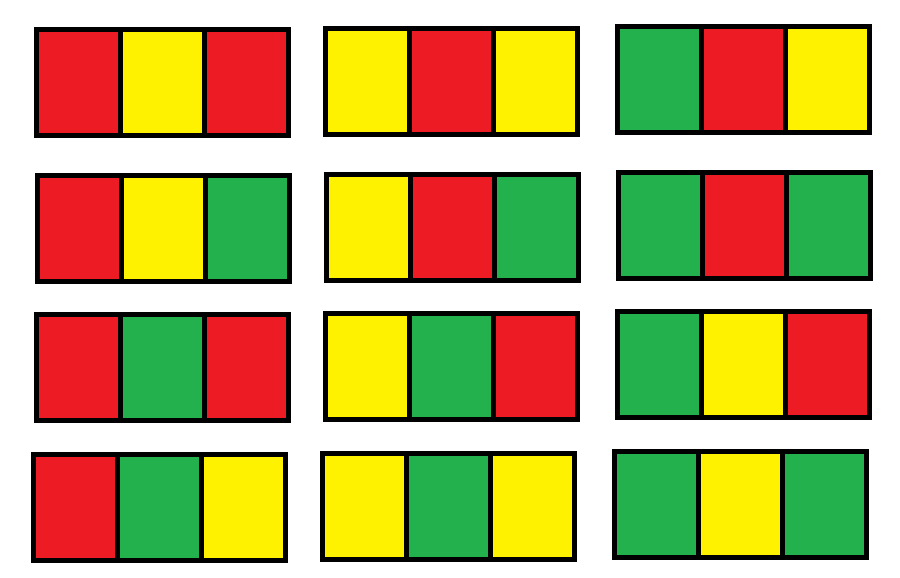
You have a grid of size n x 3 and you want to paint each cell of the grid with exactly one of the three colors: Red, Yellow, or Green while making sure that no two adjacent cells have the same color (i.e., no two cells that share vertical or horizontal sides have the same color).

Given n the number of rows of the grid, return the number of ways you can paint this grid. As the answer may grow large, the answer must be computed modulo 109 + 7.

 

Example 1:

Input: n = 1
Output: 12
Explanation: There are 12 possible way to paint the grid as shown.

Example 2:

Input: n = 5000
Output: 30228214

 

Constraints:

• n == grid.length
• 1 <= n <= 5000

Related Topics

[Dynamic Programming]

Similar Questions

1. Painting a Grid With Three Different Colors (Hard)

Hints

Hint 1

We will use Dynamic programming approach. we will try all possible configuration.

Hint 2

Let dp[idx][prev1col][prev2col][prev3col] be the number of ways to color the rows of the grid from idx to n-1 keeping in mind that the previous row (idx - 1) has colors prev1col, prev2col and prev3col. Build the dp array to get the answer.